Math Problems Vectors
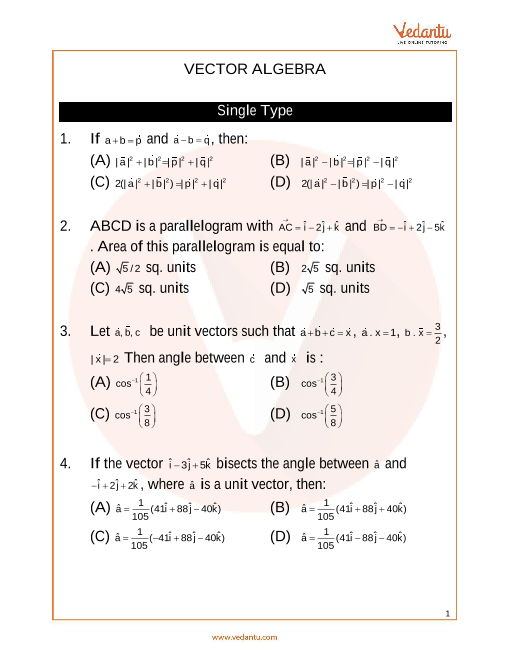
Math Problems Vectors, Indeed recently has been hunted by consumers around us, perhaps one of you personally. People now are accustomed to using the internet in gadgets to view video and image information for inspiration, and according to the name of this article I will discuss about
If the posting of this site is beneficial to our suport by spreading article posts of this site to social media marketing accounts which you have such as for example Facebook, Instagram and others or can also bookmark this blog page.
First convert from polar to cartesian to 2 decimals.

Games free fire install. Find the vector a 2 y z so that a b and a c where b 1 4 2 and c 3 3 1 vector equation. Find the sum of the vectors. Position vectors are vectors giving the position of a point relative to a fixed point the origin.
Math exercises on vectors linearly dependent vectors parallel and perpendicular vectors. If so find the coefficient of collinearity k. Displaystyle overrightarrow v 35 v 35.
Express the result in degrees. V 3 5. Subtracting a vector is the same as adding its inverse.
U 2 1. Find the scalar product. Consider three vectors u 15 v 2738 w 426.
Vector describes the movement of an object from one point to another. A b c. You can draw in the origin wherever you want.
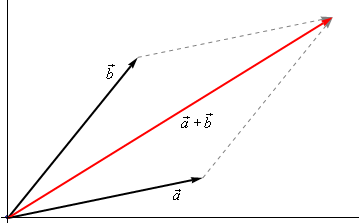
A b is the same as a b. When 2 vectors are added or subtracted the vector produced is called the resultant. Vector math can be geometrically picturised by the directed line segment.
Find the coordinates of vectors. Solving problems with vectors we can use vectors to solve many problems involving physical quantities such as velocity speed weight work and so on. The vectors are defined as an object containing both magnitude and direction.
To add two vectors you apply the first vector and then the second. Lets v 1 2 1 u 0 1 3 and w 1 0 7. The velocity of moving object is modeled by a vector whose direction is the direction of motion and whose magnitude is the speed.
X r cos o 120 cos450 120 07071 8485. X r cos o 200 cos600 200 05 100. The resultant is identified by a double arrowhead.
Displaystyle overrightarrow u 2 1 u 21 and. Y r sino 200 sin600 200 08660 17321. Find the coordinates of vectors v 2v 25v and draw the vectors in the coordinate system if.
Decide whether points a 2 5 b 4 3 and c 16 1 lie on the same line. Let us add the two vectors head to tail. Find out whether the given vectors are linearly dependent collinear.